Proofs
Carl Auer and the Ethics of the Pythagoreans
Heinz von Förster
I am, of course, delighted that Carl Auer, this extraordinary, strange and extremely creative man, is at last being commemorated by this Festschrift. I am particularly grateful to the editors of this Festschrift for giving me the opportunity to present Carl Auer with my thanks for the strong influence he had on me in his early youth. Let me tell you how I met and got to know Carl Auer. In my youth the Austrian school system was organized in such a way that all children started primary school (“Volksschule”) at the age of five or six and stayed there for five years. After finishing primary school they moved up to the secondary school (“Mittelschule”) for a further eight years, after which they could attend college, either university or technical college. I started primary school at about five years of age and spent the first four years at a state school. I was one of the worst pupils. To be more precise, I was not only one of the worst pupils. I was maybe even the worst pupil. I well remember having to sit at the very last desk. In front of me, at the last desk but one, sat two of the many orphans who came from an institution in Vienna where orphans were interned. Every morning a few minutes before eight they came marching along in their striped – horizontally striped – prison clothes. Their heads were shaved so that not even a few lice could stray onto or into their heads. Dieter Medwed, one of these pupils, sat in front of me, and he really didn‘t know the answer to one question. Yet I still had to sit behind him, for sometimes I knew an answer, but it was generally considered to be “impudent”. On these occasions the teacher, Mr. Holzinger, would come towards me smiling kindly. He would twist the hair growing behind and in front of my ear together and pull me up by this hair, so that I had to climb onto the bench and then onto the desk to make me understand my impudence.
I didn‘t feel very happy in this school, so my parents decided to transfer me from primary school to secondary school a little earlier than normal. This was possible, but one had to take an examination if one wished to go to secondary school after just four years in primary school. The problem was, however, how could I, such a bad pupil, survive or pass this exam, the centerpiece of which was in
mathematics. As I wasn‘t really that bad at math, my parents thought a few hours extra tuition would enable me to cope with the curriculum required for the exam. Thus it was suggested that a young man come to us as private tutor. His name was Carl Auer. He was recommended to my family as he was said to have been a very bad pupil too. He had failed several times and had had to repeat a year or two, but he was known to be very good in mathematics and physics. So it was that Carl Auer came to our house and began to prepare me for secondary school.
I had a lot of fun with this young man who not only knew a lot, but was also very interested in what interested me. I remember well that I was particularly interested in a certain mathematical step: the idea of proof. Carl was particularly skillful in introducing me to the “proof.” We began with my having to prove the Pythagorean theorem. Carl introduced me to this proof by demonstrating the proof Socrates showed Menon. As you may remember, Pythagoras’ theorem consists in proving that in a right triangle the sum of the squares above the two legs, a, b, namely a2 + b2, is equal in area to the square which can be drawn above the hypotenuse: c2. This results in Pythagoras’ theorem: a2 + b2 = c2.
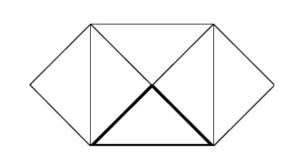
Socrates’ proof consists in drawing squares above the hypotenuse and over the two legs in an isosceles right triangle (see Fig. 1), and
showing that the area of the two small squares (each consisting of two isosceles right triangles) corresponds exactly to the area of the large square over the hypothenuse (consisting of four isosceles right triangles). I was so fascinated by this illustrative proof because I
understood what it means when one ‘sees’ something: the idea of insight. One suddenly says: Aha, now I see how these relations come about. When you have understood this proof of Pythagoras’ theorem for an isosceles right-triangle it is no longer difficult to understand this method of proof or a similar one for right triangles in general.
Fig. 1
Carl Auer soon realized that this young boy had easy access to graphic and geometrical problems and that this shouldn’t be exploited
too far, but he should rather be given insight into algebraic relations. And so he taught me that, apart from these fascinating geometrical relations between a2 and b2 and c2, there are also interesting algebraic relations. That, for example, there are whole numbers such as 3, 4 and 5 that have Pythagorean relations, namely: 32 + 42 = 52. And that this relation not only exists for 3, 4 and 5, but for many other triples, such as 5, 12 and 13 or 7, 24 and 25, which all have in common that the sum of the square of the first two numbers equals the square of the third.
These mathematical somersaults were great fun. And when the time came for the examination I passed with no difficulty at all.
A few weeks later I was admitted to the secondary school. As was the custom at that time, each new pupil was introduced to the
headmaster of the school. So I went in too, and the headmaster said: “You passed the math exam very nicely. We are happy to have you here. Who taught you math?” Proudly I replied: “Mr. Carl Auer.” At that the headmaster immediately slapped me. He grabbed me by the ear, lead me out of the room and showed me the school’s large yard. From there one could see that almost everywhere streaks of black ink had been smeared over the stone walls of the building from the windows of the third floor. And he hinted that these ink spots had been made by Carl Auer when he was a pupil of this school many years ago. He remarked: “Should you have learned characteristics other than those of a mathematician from your Carl Auer, I would advise you to get rid of them as soon as possible.”
In spite of the headmaster’s warning I continued my relationship with Carl Auer and went to see him evenings, or he came to us. We
spoke about mathematical proofs of other problems.
One day I asked him: “Tell me, Carl, you introduced me to Pythagorean numbers, where you square two natural numbers and the sum of these two corresponds to the square of a third number. Are there higher powers, so that, for example, not only a2 plus b2 equals c2, but maybe a3 plus b3 equals c3. Are there any numbers, a, b, c, which fulfill this condition?”
He became very serious after I had asked this question. He kept absolutely quiet, then after a few minutes he said: “You have touched on a big problem that a great mathematician postulated 200 years ago. His name was Fermat. Fermat gave this problem a lot of thought and finally came to the conclusion that there are no such numbers. He reported the fact that he had come to this conclusion in the margin of a book in which this problem was posed. It must have been a text book on the theory of numbers in which Fermat wrote in the margin: “I can prove that for no exponents, n, larger than two are there three numbers an + bn = cn so that this relation is valid for whole numbers. Unfortunately though the width of this margin is too narrow for me to set out the whole proof on this page.”
During the 200 years since Fermat touched on this problem – it is generally referred to as “Fermat’s Last Theorem” – ever more mathematicians have tried to work out this proof for themselves, but no one has ever succeeded. Such unsuccessful efforts of proof are, of course, a fascinating stimulus. And I asked myself straight away: How can Fermat’s theorem be proved? And I asked Carl too:
“Now tell me, have you ever tried to prove this theorem?” For a long time he said nothing. Then he nodded and said: “Yes, I have tried.” – “And, have you proved it?” – “Yes,” he said, “I’ve proved it.” – “Then why don’t you publish it, for heaven’s sake? Then all the mathematicians who have tried so hard to prove this theorem would be relieved at last!” – “Yes, I’ve thought about it a lot,” Carl answered, “but I have decided not to publish it.” – “But why not?” – “The proof could be written in the narrow margin of this paper.” – “But that’s . . . . Why don’t you publish that too?” – “Fermat knew that too, of course,” Carl replied, “and if he didn’t want to write this proof in the margin of that page, then he must have known why this proof should not be written down. Who am I, Carl Auer, to write this proof down when Fermat decided not to?” This ethical attitude of Carl Auer’s impressed me deeply. Not until much later did I realize that it in fact corresponds to that of the Pythagoreans, who did not wish to impart their deeper insights to others unless they were sure they could not lead to social abuse. One not only finds this ethical position implicit in constructivism, but also in Ludwig Wittgenstein’s Tractatus Logico-Philosophicus. Proposition 6.421 reads: ”It is clear that ethics cannot be articulated” (“Es ist klar,
daß sich Ethik nicht aussprechen läßt”). That is, that at the very moment when one starts to talk “ethics”, one begins to moralize. Then one always says how the other should behave: “Thou shalt” or “Thou shalt not!” But ethics is concerned with one’s own behaviour, one can apply it only to oneself, i.e., you say to yourself: “I should” or “I should not!” That cannot be said to anyone else, you can only say it to yourself. Just as one can prove Fermat’s theory for oneself, just as one can have many insights for oneself. The only thing one can do as a constructivist is to give others the opportunity to construct their own world. I think I owe these insights to Carl Auer’s understanding, comprehensible and mild way of talking to me when I was a small boy about mathematics and its insights.
Beweise
Carl Auer und die Ethik der Pythagoräer
Heinz von Förster
Ich bin natürlich entzückt, daß schließlich und endlich diesem außerordentlichen, diesem seltsamen und äußerst kreativen Menschen, Carl Auer, durch diese Festschrift ein Denkmal gesetzt wird. Ich bin auch den Herausgebern dieser Festschrift besonders dankbar, daß sie es mir erlauben, meinen Dank an Carl Auer für seinen großen Einfluß, den er auf mich in seiner frühen Jugend gehabt hat, abzustatten. Lassen Sie mich kurz erzählen, in welcher Weise ich Carl Auer getroffen und kennengelernt habe.
In meiner Jugend war das österreichische Schulsystem so organisiert, daß alle Kinder so mit etwa fünf oder sechs Jahren in die Volksschule kamen, dort fünf Jahre lernten, nach Absolvieren der Volksschule dann in die Mittelschule übergeführt wurden, dort acht Jahre weiterlernten, bis sie dann, nach Beenden der Schule, auf die Hochschule gehen konnten, und zwar entweder auf die Universität oder auf die Technische Hochschule. Ich trat mit etwa fünf Jahren in die öffentliche Volksschule ein und verbrachte dort meine ersten vier Jahre. Ich war einer der schlechtesten Schüler. Genau gesagt, ich war nicht einer der schlechtesten Schüler, ich war vielleicht sogar der schlechteste Schüler. Ich erinnere mich noch sehr gut, wie ich in der allerletzten Bank sitzen mußte; vor mir in der vorletzten Bank saßen zwei der vielen Waisenkinder, die damals von einem Institut in Wien interniert worden waren. Die Kinder kamen an jedem Morgen ein paar Minuten vor acht in ihren gestreiften – quergestreiften – Sträflingsanzügen und mit ihren kahlgeschorenen Köpfen, damit nun ja nur nicht ein paar Läuse sich auf diese Köpfe verirren könnten, anmarschiert. Dieter Medwed, einer von diesen Schülern, saß vor mir. Er wußte wirklich auf keine Frage eine Antwort. Ich mußte aber hinter ihm sitzen, denn manchmal wußte ich zwar eine Antwort, die wurde aber gewöhnlich als ‘frech‘ beurteilt. Und so kam dann der Lehrer
Holzinger auf mich zu, freundlich lächelnd, drehte die Haare, die hinter meinem Ohr und vor meinem Ohr wuchsen, zusammen und zog mich an diesen Haaren langsam hoch, so daß ich zuerst die Bank und dann den Schreibtisch hinaufklettern mußte, um meine Frechheit zu verstehen.
Ich fühlte mich nicht sehr wohl in dieser Schule und so beschlossen meine Eltern, mich schon etwas früher aus der Volksschule in das Gymnasium überwechseln zu lassen. Das war möglich, aber man mußte eine Offenbarungsprüfung machen, wenn man nur vier Jahre in der Volksschule zugebracht hatte und dann schon ins Gymnasium gehen wollte. Das Problem war aber, wie ich, dieser schlechte Schüler, diese Offenbarungsprüfung überstehen oder bestehen konnte, deren Hauptteil eine Mathematikprüfung war. So dachten meine Eltern, da ich ja gar nicht so schlecht in Mathematik war, mir ein paar Nachhilfestunden geben zu lassen, so daß ich das Pensum, welches von mir verlangt wurde, tatsächlich bei dieser Offenbarungsprüfung bewältigen konnte. Mein Vater hatte gute Beziehungen zur Industrie, auch zur chemischen Industrie. Und so wurde vorgeschlagen, mir einen jungen Mann als einen Hauslehrer zukommen zu lassen: sein Name war Carl Auer. Er wurde deshalb unserer Familie empfohlen, weil er auch ein sehr schlechter Schüler gewesen sein soll. Er war in mehreren Fällen durchgefallen, hatte eine oder zwei Klassen wiederholen müssen, aber war bekannt dafür, in Mathematik und in Physik gut zu sein. So kam Carl Auer in unser Haus und begann, mich auf das Gymnasium vorzubereiten.
Ich hatte großen Spaß mit diesem jungen Menschen, der wirklich sehr viel wußte und sich sehr für meine Neigungen interessierte. Ich kann mich noch sehr gut erinnern, daß mich ein bestimmter Schritt in der Mathematik ausgesprochen interessierte: nämlich der „Beweis“. Carl hatte eine besonders geschickte Weise, mich in diese Beweisidee einzuführen. Und zwar haben wir damit angefangen, daß ich den Pythagoräischen Lehrsatz beweisen sollte. Natürlich er hat mich in diese Beweistheorie damit eingeführt, daß er mir den Sokratischen Beweis, den Sokrates mit Menon auch durchgeführt hat, vorführte. Wie Sie sich erinnern, besteht dieser Pythagoräische Lehrsatz darin, daß man beweist, daß in einem rechtwinkligen Dreieck die Summe der Quadrate über den beiden Katheten, nämlich a2 + b2, flächengleich ist mit dem Quadrat über der Hypothenuse, c2. Das gibt den berühmten Pythagoräischen Lehrsatz: a2 + b2 = c2.
Der Sokratische Beweis besteht darin, daß man Quadrate über der Hypothenuse und den beiden Katheten eines gleichschenkligen und rechtwinkligen Dreiecks (siehe Abb. 1) zeichnet und damit aufzeigt, daß die Fläche der zwei kleinen Quadrate (jedes bestehend aus zwei gleichschenkligen und rechtwinkligen Dreiecken) exakt mit der Fläche des großen Quadrats über der Hypothenuse (bestehend aus vier gleichschenkligen und rechtwinkligen Dreiecken) korrespondiert. Mich hat dieser anschauliche Beweis so fasziniert, weil ich verstanden habe, was es bedeutet, wenn man etwas einsieht: die Idee der Einsicht. Man sagt plötzlich: Aha, jetzt sehe ich, wie es zu diesen Relationen kommt. Natürlich ist es dann auch gar nicht schwer, wenn man diese Einsicht des Pythagoräischen Lehrsatzes für ein rechtwinkliges Dreieck gesehen hat, daß man dann diese oder eine verwandte Beweismethode für allgemeine Dreiecke auch einsehen kann.
Fig. 1

Bald hat Carl Auer gesehen, daß dieser kleine Bub, der ich war, einen leichten Zugang zu anschaulichen und geometrischen Problemen hat. Er wollte mir nun auch Einsicht in die algebraischen Relationen beibringen. So hat er mir sehr bald beigebracht, daß es neben diesen geometrisch faszinierenden Relationen zwischen a2, b2 und c2 auch algebraisch interessante Relationen gibt. Er hat mir erklärt, daß es ganze Zahlen, wie z. B. 3, 4 und 5 gibt, die die Pythagoräische Relation haben, nämlich 32 + 42 = 52, und daß es diese Beziehung außerdem auch zwischen vielen anderen, wie z. B. 5, 12 und 13 oder 7, 24 und 25 gibt. Für alle diese Dreiergruppen gilt, daß die Summe der Quadrate der ersten beiden, dem Quadrat der dritten Zahl gleich ist.
Ich hatte großen Spaß bei diesen mathematischen Purzelbäumen. Und wie es nun schließlich zur Prüfung kam, habe ich sie ohne Schwierigkeiten bestanden.
Ein paar Wochen später wurde ich daher zum akademischen Gymnasium zugelassen. Und wie es damals üblich war, wurde jeder neue Ankömmling dem Direktor vom Gymnasium vorgestellt. So kam auch ich herein, und der Direktor hat gesagt: „Du hast sehr nett diese Mathematikprüfung bestanden. Wir freuen uns, dich hier zu haben. Wer hat dir denn die Mathematik beigebracht?“ Stolz sagte ich: „Das war ein Herr Carl Auer.“ Worauf ich sofort eine Ohrfeige vom Direktor bekam. Er packte mich am Ohr, führte mich aus dem Zimmer heraus und zeigte mir den großen Hof des Gymnasiums. Dort konnte man sehen, daß von den Fenstern des dritten Stocks aus fast überall schwarze Tintenstriche über die Steinmauern des Gebäudes geschmiert waren. Und er deutete an, daß diese schwarzen Tintenflecke von Carl Auer gemacht wurden, als er vor vielen Jahren ein Schüler in dieser Schule war. Er bemerkte:
Solltest du andere Eigenschaften als die eines Mathematikers von deinem Carl Auer gelernt haben, dann würde ich sehr empfehlen, diese Eigenschaften so schnell als möglich abzulegen.“
Trotz der Warnung des Direktors habe ich mein Verhältnis mit Carl Auer noch weiter fortgesetzt und ging abends zu ihm oder er kam zu uns. Dann haben wir uns über mathematische Beweise anderer Probleme unterhalten.
Eines schönen Tages fragte ich ihn: „Sag einmal, Carl, du hast mir diese Pythagoräischen Zahlen beigebracht, bei denen die Summe der Quadrate zwei natürlicher Zahlen dem Quadrat einer dritten Zahl entspricht. Gibt es höhere Potenzen, wie z. B. a3 + b3 = c3, bei denen drei natürliche Zahlen, a, b, c, diese Bedingung erfüllen?“
Er wurde ganz ernst, nachdem ich ihn diese Frage gefragt hatte. Er war sogar ganz still und nach einigen Minuten sagte er: „Du hast
ein ganz großes Problem berührt, das ein großer Mathematiker vor 200 Jahren schon einmal postuliert hat. Das war Fermat. Fermat hat sich über dieses Problem lange den Kopf zerbrochen und ist schließlich zu dem Schluß gekommen, daß es keine solchen Zahlen gibt. Und zwar hat er den Bericht, daß er zu diesem Schluß gekommen ist, am Rand eines Buches hingeschrieben, in dem dieses Problem aufgeworfen wurde. Das muß wohl ein Lehrbuch der Zahlentheorie gewesen sein. Dort schrieb Fermat auf den Rand: ’Ich kann zeigen, daß es für keinen Exponenten, der größer ist als zwei, drei natürliche Zahlen gibt, die die Beziehung an + bn = cn erfüllen. Leider aber ist die Breite des Papierrandes hier zu schmal, daß ich den vollen Beweis auf dieser Seite ausführen könnte.“
In den 200 Jahren, seitdem Fermat dieses Problem berührte – es wird gewöhnlich als Fermats letztes Theorem bezeichnet – haben Mathematiker und Mathematiker sich bemüht, diesen Beweis für sich selbst zu entwickeln, aber es ist niemandem gelungen. Solche ungeglückten Beweisversuche sind natürlich faszinierende Stimuli. Und ich habe mich natürlich sofort gefragt: Wie kann man diesen Fermatschen Satz beweisen. So hab ich Carl gefragt:
„Na, sag einmal, hast du dich schon einmal bemüht, diesen Satz zu beweisen?“ Da ist er lange stumm geblieben, dann hat er genickt
und gesagt: „Ja, ich habe mich bemüht.“ – „Na und, hast du ihn bewiesen?“ – „Ja“, sagte er, „ich habe ihn bewiesen.“ – „Ja, um Himmelswillen, warum publizierst du das nicht, dann würden doch diese vielen Mathematiker, die sich so bemühen, diesen Satz zu beweisen, endlich erlöst werden?“ – „Ja, ich habe mir das sehr überlegt“, antwortete Carl, „aber ich hab mich entschlossen, das nicht zu publizieren.“ – „Ja, warum denn?“ – „Ja, der Beweis läßt sich auf den Rand, auf den schmalen Rand dieses Papieres schreiben.“ – „Ja, das ist doch, warum veröffentlichst du das nicht auch?“ – „Ja, aber Fermat hat das natürlich auch gewußt, und wenn er diesen Beweis nicht auf den Rand des Papieres schreiben wollte, dann hat er wohl sehr gut gewußt, warum dieser Beweis nicht geschrieben werden soll. Woher soll ich, Carl Auer, dann diesen Beweis schreiben, wenn Fermat sich entschlossen hat, ihn nicht zu schreiben?“
Diese ethische Haltung Carl Auers hat mich tief beeindruckt. Und viel später habe ich dann gesehen, daß sie eigentlich der der Pythagoräer entspricht, die ja auch nicht ihre tieferen Einsichten anderen mitteilen wollten, nämlich anderen, von denen sie nicht sicher waren, ob sie diese Einsichten, nicht zu einem Mißbrauch (auch einem sozialen Mißbrauch) verwenden würden. Diese ethische Haltung ist implizit nicht nur im Konstruktivismus zu finden, sondern auch in Ludwig Wittgensteins „Tractatus Logico-Philosophicus”. Satz 6.421: „Es ist klar, daß sich die Ethik nicht aussprechen läßt“. Das heißt, im Moment, wo man über Ethik sprechen will, beginnt man ja schon zu moralisieren. Dann spricht man immer, wie sich der andere zu verhalten hat: Du sollst oder du sollst nicht. Aber die Ethik bezieht sich ja auf das eigene Verhalten, d. h. man sagt zu sich selbst: Ich soll oder ich soll nicht. Das kann nicht zu anderen gesagt werden, das kann man nur zu sich selbst sagen. So, wie man das Fermatsche Prinzip für sich selber beweisen kann, so wie man für sich selber viele andere Einsichten haben kann. Das einzige, was man machen kann als ein Konstruktivist, ist anderen die Gelegenheit zu geben, ihre eigene Welt zu konstruieren. Diese Einsichten verdanke ich, glaube ich, Carl Auers verständnisvoller, einsichtsvoller und milder Art, mit mir als kleinem Buben über die Mathematik und ihre Einsichten zu sprechen.
